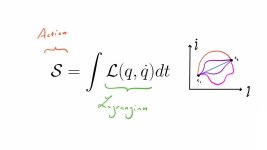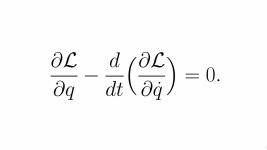Learning about the Lagrangian and path of least action

[repoman]

This is some cool stuff that spans classical and quantum mechanics.

I watched a couple excellent derivations of the Euler-Lagrange equation and how it applies to action then I played around with a simple example.

Take a projectile shot up in the air with initial velocity, v₀ and follow it until it stop and is ready to fall back. The Action integral for this path was -1/6 m(v₀ )³/g which is in units of J-s or energy*time.

Did the same for having the projectile reach the vertex point in the same time but with a constant velocity and got Action = -1/8 m(v₀ )³/g which is larger.

Then had the projectile go twice as fast and then stay stationary for the rest of the time (like a taxi meter running). The action in this case was + 3/8 m(v₀ )³/g

It is kind of hard to wrap my head around this, but it starting to make sense.


Also, what is the physical significance to units of E*t (energy*time) being the same as P*x (momentum*position)?

 

[steve_bank]

I am not familiar with the equation and what it means.

In general energy is a scalar quantity in Joules.

I'd think the energy of a projectile at any instant is kinetic energy + gravitational potential energy.

Rate of energy is Joules/Second = Watts, power.
1 Watt = 1 J/s.

The integral of force * distance, Newtons * Meters is work. Work and energy and heat are equivalent with the common unit Joules.

The work integral is path independent. No matter the path you walk up a hill, the work is the same. You can google it.

 

[repoman]

Curve 2 is the path that actually happens and has the lowest value of "action" often denoted as S.

 

[steve_bank]

Feyman
https://www.feynmanlectures.caltech.edu/II_19.html

https://en.wikipedia.org/wiki/Stationary_Action_Principle

The stationary action principle – also known as the principle of least action – is a variational principle that, when applied to the action of a mechanical system, yields the equations of motion for that system. The principle states that the trajectories (i.e. the solutions of the equations of motion) are stationary points of the system's action functional. The term "least action" is a historical misnomer since the principle has no minimality requirement: the value of the action functional need not be minimal (even locally) on the trajectories.[1]

The principle can be used to derive Newtonian, Lagrangian and Hamiltonian equations of motion, and even general relativity (see Einstein–Hilbert action). In relativity, a different action must be minimized or maximized.

The classical mechanics and electromagnetic expressions are a consequence of quantum mechanics. The stationary action method helped in the development of quantum mechanics.[2] In 1933, the physicist Paul Dirac demonstrated how this principle can be used in quantum calculations by discerning the quantum mechanical underpinning of the principle in the quantum interference of amplitudes.[3] Subsequently Julian Schwinger and Richard Feynman independently applied this principle in quantum electrodynamics.[4][5]

The principle remains central in modern physics and mathematics, being applied in thermodynamics,[6] fluid mechanics,[7] the theory of relativity, quantum mechanics,[8] particle physics, and string theory[9] and is a focus of modern mathematical investigation in Morse theory. Maupertuis' principle and Hamilton's principle exemplify the principle of stationary action.

The action principle is preceded by earlier ideas in optics. In ancient Greece, Euclid wrote in his Catoptrica that, for the path of light reflecting from a mirror, the angle of incidence equals the angle of reflection.[10] Hero of Alexandria later showed that this path was the shortest length and least time.[11]

Scholars often credit Pierre Louis Maupertuis for formulating the principle of least action because he wrote about it in 1744[12] and 1746.[13] However, Leonhard Euler discussed the principle in 1744,[14] and evidence shows that Gottfried Leibniz preceded both by 39 years.[15][16][17]

 

[steve_bank]

I started working through the lecture. Theoretical math usually does not interest ,e. nut this does. I was unaware of the importance.


This brings the problem into focus and provides context for met.

“Problem: Find the true path. Where is it? One way, of course, is to calculate the action for millions and millions of paths and look at which one is lowest. When you find the lowest one, that’s the true path.

“That’s a possible way. But we can do it better than that. When we have a quantity which has a minimum—for instance, in an ordinary function like the temperature—one of the properties of the minimum is that if we go away from the minimum in the first order, the deviation of the function from its minimum value is only second order. At any place else on the curve, if we move a small distance the value of the function changes also in the first order. But at a minimum, a tiny motion away makes, in the first approximation, no difference (Fig. 19–8).

“There are many problems in this kind of mathematics. For example, the circle is usually defined as the locus of all points at a constant distance from a fixed point, but another way of defining a circle is this: a circle is that curve of given length which encloses the biggest area. Any other curve encloses less area for a given perimeter than the circle does. So if we give the problem: find that curve which encloses the greatest area for a given perimeter, we would have a problem of the calculus of variations—a different kind of calculus than you’re used to.