Tigers!
Veteran Member
- Joined
- Sep 19, 2005
- Messages
- 4,367
- Location
- On the wing, waiting for a kick.
- Basic Beliefs
- Bible believing revelational redemptionist (Baptist)
I occasionally tutor school students at our church in algebra, trig, logarithms, exponents etc. Very rarely do I drift into calculus.
However, a girl and I got to talking about the classis problem of boring a tunnel from one side of the earth to the other through the centre. We discussed it's SMH (Simple Harmonic Motion) behaviour under the influence of just gravity. She asked would SMH would occur if a chord was bored through the earth from, say, Australia to England and a mass dropped in said chord? I had never considered that before and have spent a few weeks thinking about it. I have reached the end of my mathematical ability.
With reference to the attached picture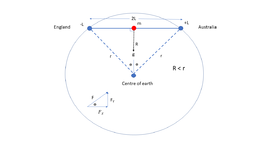
The path of the particle is considered to be on the X-axis and gravity is pointed towards the centre of the earth and is thus -Y. The length of the path is 2L with range of -L to +L. The centre of the path can be considered to be the origin at (0,0). The motion of the particle is SMH. The total angle covered by the path is 2ɵ.
Some equations are
Fx = g cosɵ = max = m.d2x/dt2
Fy = g sinɵ = may = m. d2y/dt2
I could convert into polar form.
Main question:
Could g acting on a particle at angle ɵ give rise to SMH?
Next question:
If so, what are the next steps to solve? Has this been considered somewhere by someone that I could look at?
However, a girl and I got to talking about the classis problem of boring a tunnel from one side of the earth to the other through the centre. We discussed it's SMH (Simple Harmonic Motion) behaviour under the influence of just gravity. She asked would SMH would occur if a chord was bored through the earth from, say, Australia to England and a mass dropped in said chord? I had never considered that before and have spent a few weeks thinking about it. I have reached the end of my mathematical ability.
With reference to the attached picture

The path of the particle is considered to be on the X-axis and gravity is pointed towards the centre of the earth and is thus -Y. The length of the path is 2L with range of -L to +L. The centre of the path can be considered to be the origin at (0,0). The motion of the particle is SMH. The total angle covered by the path is 2ɵ.
Some equations are
Fx = g cosɵ = max = m.d2x/dt2
Fy = g sinɵ = may = m. d2y/dt2
I could convert into polar form.
Main question:
Could g acting on a particle at angle ɵ give rise to SMH?
Next question:
If so, what are the next steps to solve? Has this been considered somewhere by someone that I could look at?
