• Welcome to the new Internet Infidels Discussion Board, formerly Talk Freethought.

The Einstein Tile

Jarhyn said:
Elixir said:
LOVE IT!
Click to expand...
Apparently there is a whole family of them, some members with as few as 6 sides.
Click to expand...
The subject of Einstein shapes also coincidentally has, as far as I know, a relationship to the idea of the quasicrystal.

This begs the question: is there an Einstein monovolume?
 
Jarhyn said:
is there an Einstein monovolume?
Click to expand...
I would not know the first thing about how to go about trying to find one, but I wish you luck! When you find it I’ll be all “I knew him when…”
 
Elixir said:
Jarhyn said:
is there an Einstein monovolume?
Click to expand...
I would not know the first thing about how to go about trying to find one, but I wish you luck! When you find it I’ll be all “I knew him when…”
Click to expand...
Lol no im not that insane.

Someone did this with cards to make sides, I would tell a machine to do it with polyhedra.

There's a change in magnitude and complexity when working in 3 dimensions, too.

I just thought it was interesting math news.

It's a logical question to ask.
 
Jarhyn said:
Elixir said:
Jarhyn said:
is there an Einstein monovolume?
Click to expand...
I would not know the first thing about how to go about trying to find one, but I wish you luck! When you find it I’ll be all “I knew him when…”
Click to expand...
Lol no im not that insane.
Click to expand...

Apparently, this is what quasicrystals are. It's interesting that nature proved the 3d corollaries of quasicrystalline systems to the aperiodic tilesets.

I was so fascinated, that I decided to look up some things about them. As long as you align them correctly, any group can be forced to bond to some subset of any other group, and will change conformity to what is needed to match according to some region of the infinite aperiodic normal volume.

Its a journal specifically about putting them together, and what happens when you try it.
 
I don't know why, but this totally hits my brain's funny bone. Just looking at the sample of "the hat" makes me laugh.
The animation is a real headfuck.
 
Elixir said:
I don't know why, but this totally hits my brain's funny bone. Just looking at the sample of "the hat" makes me laugh.
The animation is a real headfuck.
Click to expand...
If you pay close attention, one of the animation frames reveals a simple Chevron as a valid aperiodic monotile shape.

I think maybe we didn't know how to prove aperiodicity was an available configuration that chevrons could be placed in.
 
Jarhyn said:
If you pay close attention, one of the animation frames reveals a simple Chevron as a valid aperiodic monotile shape.
Click to expand...
I caught that first time through and wasn't sure until I watched it through again. That's a real mindblower too.
I guess it's the idea of infinite uniqueness arising spontaneously from so much uniformity - it's like a sample illustration of "how everything works".
 
Elixir said:
Jarhyn said:
If you pay close attention, one of the animation frames reveals a simple Chevron as a valid aperiodic monotile shape.
Click to expand...
I caught that first time through and wasn't sure until I watched it through again. That's a real mindblower too.
I guess it's the idea of infinite uniqueness arising spontaneously from so much uniformity - it's like a sample illustration of "how everything works".
Click to expand...
Yes, but somehow with more complexity to the shapes than simple width, breadth, and height, but with some surfaces electrical, and some magnetic, with some strong and some weak... Maybe in some ways just as aperiodic through time as space, except for in singular, tiny instances like the Chevron, where maybe a region can display a penchant for limited periodicity ALA the "time crystal".
 
Tigers! said:
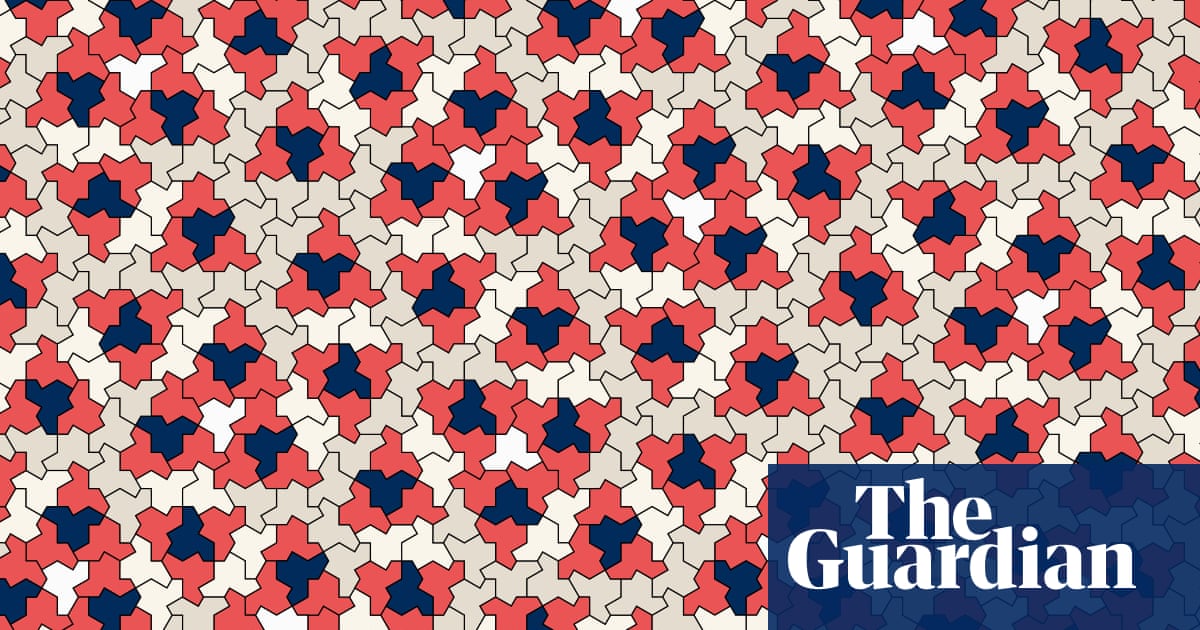
Look at it too long and my eyes go funny.
But very interesting
Click to expand...
I know. The brain questions how it can see something like an infinitely interlocking floor that never repeats. I don't even know if it's possible to incorrectly fit them together, as long as you get three to interlock.

It makes me wonder what the effect on rotational conformity would be if you were to forcibly rotate with enough energy to force the surrounding tiles to rotate until the group reached a different configuration from a given point?

Let's say I flip a *single* tile. Starting with all tiles it borders on, how will the neighboring tiles have to move to conform at the lowest total amount of group rotation?

How much rotation does it take the next layer? And the next? And the next?

How many layers will I go through before the shape conforms to the new "center tile" without needing additional rotation?

What does this look like if we explore the set of states wherein a more rotated inner layer would allow for completion? Ie, does the system adapt to absorb any such single change?

What is the minimum change size to produce a requirement to continued conformity changes?

Is there any size of conformity change that will force a systemwide modification that will continue indefinitely?

If you zoom out far enough, and treat the tile colors they use as RGB or CMYK or whatever, will you find a region that tells a story comic book style beginning to end? What would the region of the tilespace around that even look like? Would it contain other similar comic book stories? Or are there infinitely many regions that contain that comic book, some weird and some spookily sensible?

The implications of this aperiodic behavior of a tiled surface are absolutely hilarious.

Considering the Chevron system, is there some way to guarantee breaking a periodic Chevron field(s) this way?

What is the radius of any given break on the symmetrical Chevron field(s), if it's impossible to break a given periodic field indefinitely into an aperiodic one from a conformity change?

Is there any conformity change to the aperiodic hat Chevron field that will shove it into periodicity?
 
From my previous post, let's assume a concept of energy level in addition to the conformity change: let's say reconfiguration of the matrix costs energy, some for rotational conformity and some for flip-conformity. Or whatever those three colors. IDFC.

Anyway, assuming such a cost exists, what is the quantity of energy necessary to force a conformity change of any one Chevron or tile to be absorbed vs rejected as insufficient to trigger change on least action?

This may imply a renderable 2-d version of particle physics that has both periodic and aperiodic continuations, though I think the aperiodic physics would be fundamentally more interesting.
 
You must log in or register to reply here.
Back
Top Bottom