steve_bank
Diabetic retinopathy and poor eyesight. Typos ...
Demystifying QM.
This in the text I sed in a class.
A wave function is a solution to the wave equation. An easy example to visualize is the wave function for a particle in a square indite poetical well, a model for a glass laser tube.
The solution is sines and cosines. When the wavelength of a photon in a laser tube is an integer multiple of the length of the tube resonance occurs and there is sinusoidal standing EM wave in the tube or box. The wave in a laser the is physically measurable.
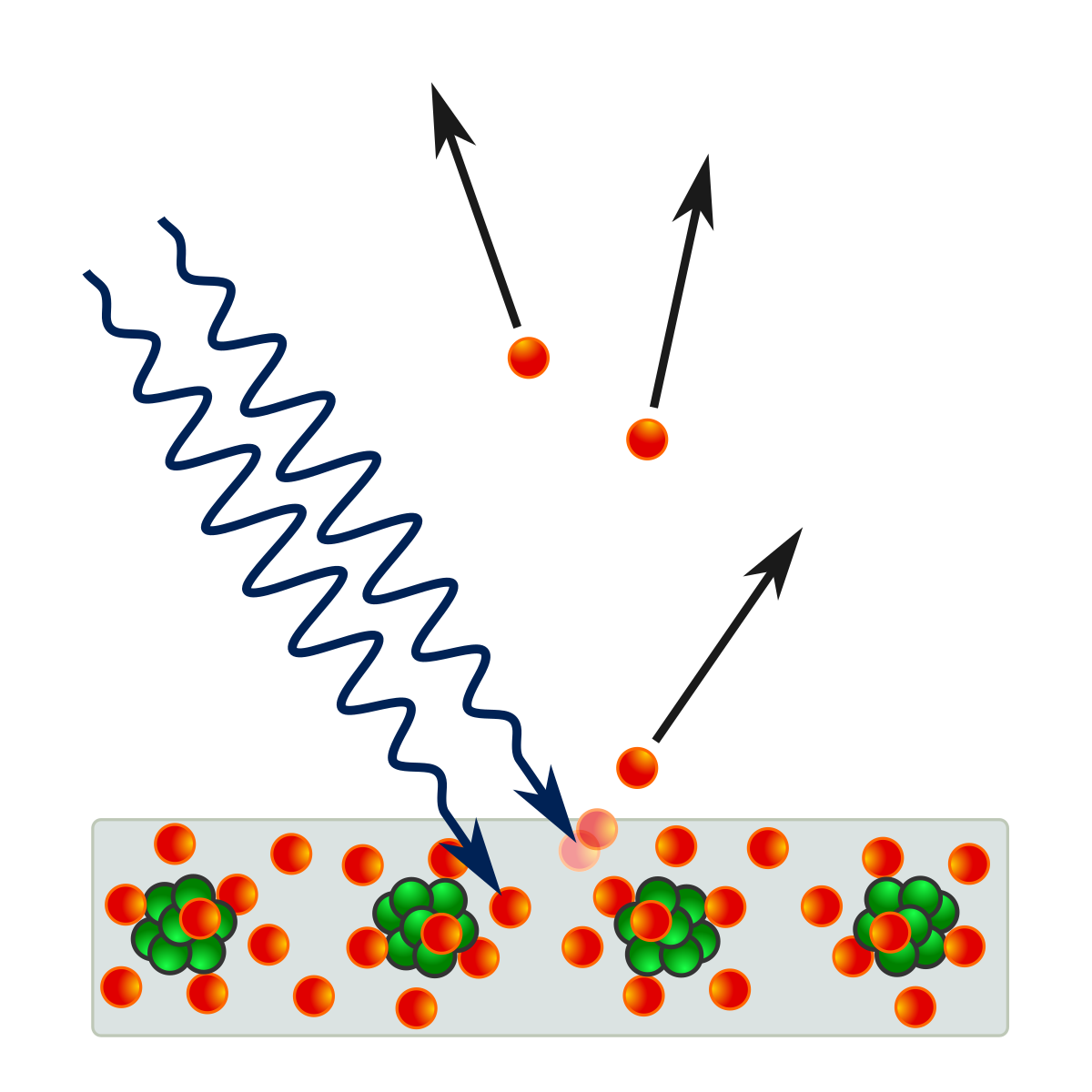
The wave function for an infinite poetical well represents the probability of a particle(photon) being in a volume as dy*dx*dz -> 0. Hence the term probability wave.

 en.wikipedia.org
en.wikipedia.org
When a measurement is made the wave function 'collapses' into one state.
 en.wikipedia.org
en.wikipedia.org
When the wave function collapses there is no debris, loud boom, or bright flash....
This in the text I sed in a class.
A wave function is a solution to the wave equation. An easy example to visualize is the wave function for a particle in a square indite poetical well, a model for a glass laser tube.
The solution is sines and cosines. When the wavelength of a photon in a laser tube is an integer multiple of the length of the tube resonance occurs and there is sinusoidal standing EM wave in the tube or box. The wave in a laser the is physically measurable.
The wave function for an infinite poetical well represents the probability of a particle(photon) being in a volume as dy*dx*dz -> 0. Hence the term probability wave.

Particle in a box - Wikipedia
When a measurement is made the wave function 'collapses' into one state.
Wave function collapse - Wikipedia
When the wave function collapses there is no debris, loud boom, or bright flash....


 At such speeds relative to what frame of reference?
At such speeds relative to what frame of reference?